Pythagorean Skating
Let's consider another case where we might want to use the Pythagorean Theorem. I'm skateboarding down a ramp. The ramp that I slide down is 20 ft long, and it's 6 ft tall. So, how far out does the ramp go?
Again, let's use our a 2 + b 2 = c 2. My a, in this case, is my height (6 ft). The b I don't know because I don't know how far I'm travelling perpendicular to my height. I do know the hypotenuse and that's 20 (I'm travelling down a 20 ft ramp). So, let's plugin 20 for c. I get 6 2 + b 2 = 20 2. I can solve this out, and I find that b is 19 ft.
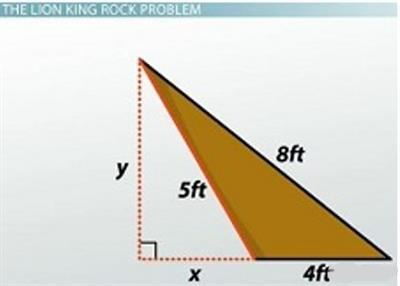
Let's say you've got a different kind of triangle. Here, you've got the Lion King peak. You want to find out how high up the Lion King peak is and how much it overhangs. If I draw this out, I'm looking for this distance (the overhang distance), as well as the height.
Let's say I know a little bit about this big rock. I know that it's 4 ft across, 5 ft this way and about 8 ft this way. How can I use the Pythagorean Theorem here? I don't actually have two right triangles, so how can I find the overhang and the height of this Lion Kingpeak? I know that I have a right triangle made up of one length that I know (the height and the overhang), but in this case I have two unknowns and only one equation. So, is there another triangle that I can use?
Let's write out our first equation. Here's our overhang that we're going to call x, the height y and this hypotenuse for this triangle is actually 5. So, I have x 2 + y 2 = 5 2. Then, I'm going to draw a second triangle and I'm still going to use the overhang height, but now I'm going to include the entire Lion King peak in this example. Now, my hypotenuse is 8. I know that one side is y, but what's the other side? What's the length of this side? It's going to be 4 + x, because x is this length here (the overhang). It's the width of my Lion King peak plus the amount that it overhangs.
Let's plug all that in: (x + 4) 2 + y 2 = 8 2. That's great. I have two equations and two unknowns. This is just an algebra problem! I'm going to solve this by subtracting my little triangle equation from my other equation. That cancels out the y terms. I end up with an equation I can simplify as 8x + 16 = 39 or x = 2.875.
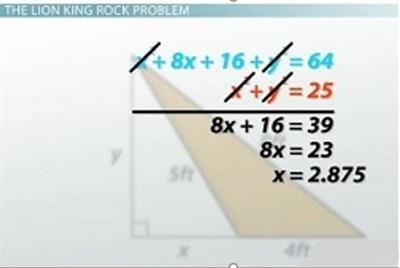
Okay, I've got x, now I just need to solve for y. I'm going to use what I know for x for the overhang, which is x 2 + y 2 = 5 2. Plug in x, square it, add y 2 (which is unknown) equals 5 2. I solve that, and I end up with y equaling just about 4.1. What I've found out is by using the Pythagorean Theorem twice on two different right triangles, my rock has a height of 4.1 ft and an overhang of about 2.9 ft.
Lesson Summary
Let's review. We use the Pythagorean Theorem with right triangles. That's where the two short lengths (a and b) are perpendicular to one another, and c is the hypotenuse. The Pythagorean Theorem says a 2 + b 2 = c 2.