Solving with Perimeter
In all of these examples, we were given the measure of each side to calculate the perimeter. Now, let's look at how we can use perimeter to find the measure of the sides.
Here's rectangle DOTS, which has a perimeter of 56:
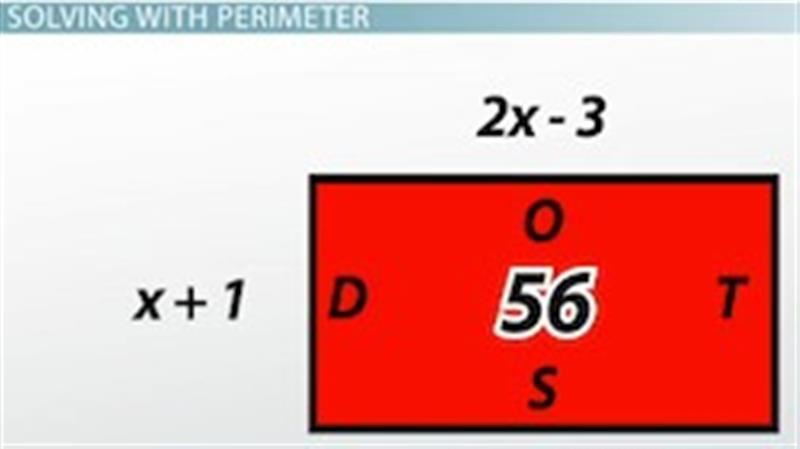
Rectangle DOTS, with a perimeter of 56
If the length of the rectangle is 2x - 3, and the width of the rectangle is x + 1, how long is the rectangle?
For this problem, knowing the value of x will allow us to determine the length. So, we must first solve for x. Since this is a rectangle, let's multiply the length by two and multiply the width by two. Then, we will add them together to equal the perimeter. This process gives us 2(2x - 3) + 2(x + 1) = 56. From distributing, we get 4x - 6 + 2x + 2 = 56, and after combining like terms, we have 6x - 4 = 56. We must now add 4 to both sides to cancel it out of the equation, leaving us with 6x = 60. Our next step will be to divide each side by 6, giving us x = 10.
Since we were only asked to determine the length of the rectangle, let's plug 10 into the equation for the length, which is 2x - 3. By substitution, we have 2(10) - 3. To calculate, we refer to the order of operations and multiply 2 * 10 before subtracting 3. In the end, the length of rectangle DOTS is 17.
Here's one last example.
Triangle PUD has a perimeter of 162 cm. Side PD is 4 less than twice a number, side PU is 8 less than the number, and side UD is 15 less than three times the number. What is the length of each side of triangle PUD? Since this problem does not directly give us the equation for each side, we will have to create them based on the descriptions.

Triangle PUD, with a perimeter of 162
The length of side PD is 4 less than twice a number. Since we don't know what this number is, we will call it x. So, PD is 4 less than two times x, which means that PD = 2x - 4. Side PU is 8 less than the number, so PU = x - 8, and side UD is 15 less than three times the number, which means that UD = 3x - 15.
We will add all three equations to equal the perimeter. So, our beginning setup is 2x - 4 + x - 8 + 3x- 15 = 162. Combining like terms takes us to 6x - 27 = 162. From here, we cancel out the 27 by adding it to both sides, leaving us with 6x = 189. Then, we will divide each side by 6 to conclude that x = 31.5. We're ready to determine all of the side lengths for triangle PUD.
Substituting 31.5 into each equation, we see that PD = 59 cm, PU = 23.5 cm, and UD = 79.5 cm. To check our work, let's add up all of these lengths. Doing so gives us 59 + 23.5 + 79.5, which is equal to 162. Since this sum is the same as the perimeter we were given, we know that we have correctly solved the problem.
Lesson Summary
In review, the perimeter is defined as the distance around an object, shape or figure and is calculated by adding the lengths of all the sides of a polygon. Rectangles have two pairs of congruent sides. The lengths have the same value, and the widths have the same value. This allows us to use addition or a combination of addition and multiplication to calculate the perimeter.
On the other hand, some triangles have congruent sides and some do not; therefore, we should only use addition to calculate the perimeter of triangles. With both shapes, we can also use the perimeter to work backward and determine the length of each side.