Points and Coordinate Pairs
It's a little bit clunky to walk around saying things like 'This is the point with an x-coordinate of 5 and a y-coordinate of 2,' so we use a shorthand to talk about it. Instead of all that stuff, you can write the numbers as an ordered pair.
An ordered pair is defined as a set of coordinates shown as two numbers inside parentheses. The x-coordinate always comes first, and the y-coordinate always comes second. So the coordinates of Point B on the graph would be (5, 2).
Here are some examples of ordered pairs.
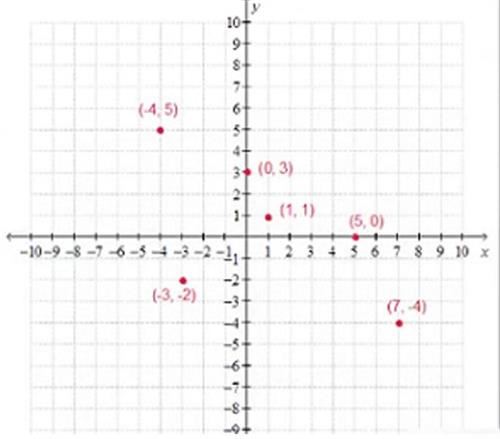
A point that's on one of the two axes has a 0 for the other coordinate. For example, this point is labeled (5, 0), because it's 5 units to the right of the middle of the graph, and 0 units up or down. This point is labeled (0, 3), because it's 3 units up from the middle of the graph, and 0 units to the right or left.
The point at the very middle of the graph is called the origin, and its coordinates are (0, 0), because it's 0 units away from the center of the graph in both directions.
If we draw a line on the graph, sometimes the line crosses either the x-axis or the y-axis. The point where a line crosses an axis is called an intercept. If it's the x-axis, it's the x-intercept; if it's the y-axis, it's the y-intercept. A line can have either an x-intercept, a y-intercept, or both.
Quadrants
If you take a step back and look at this graph, you'll see that the x and y-axes divide it into four parts.

Each of these parts is called a quadrant. To make it easy to talk about quadrants, each one gets a number, starting from the first quadrant at the top right. In the first quadrant, both x and y are positive. The top left is the second quadrant. Here, x is negative because we're left of the origin, but y is positive. The bottom left is the third quadrant, where both x and y are negative, and the bottom right is the fourth quadrant, where x is positive but y is negative.
Lesson Summary
In this lesson, you learned about the Cartesian plane, which is a type of grid that mathematicians use to show the location of lines and points. As you start doing more advanced algebra and geometry, this will help you solve all kinds of problems.
The Cartesian plane has an x-axis and a y-axis that cross each other at the origin. The x-axis is horizontal and measures distance to the left and right of the origin. The y-axis is vertical and measures distance up and down. It's basically like two number lines, with one going the usual way and another one flipped on its side to measure distance up and down.
Points on the Cartesian plane are labeled as ordered pairs, in the format (x, y), where x represents the x-coordinate and y represents the y-coordinate. If a line crosses one of the axes, the point where it crosses is called the x-intercept or y-intercept.
The Cartesian plane is divided into four quadrants, starting with the first quadrant at the top right, and moving counterclockwise.
That's a lot of terminology to learn all at once, so don't sweat it if you don't get it all immediately. As you start using Cartesian planes in actual problems, you'll pick it up really fast, and, eventually, you won't even have to think about it.
Learning Outcomes
After gaining knowledge of graphs from this lesson, you can demonstrate that knowledge by doing the following:
- Describe a Cartesian plane
- Graph an ordered pair on the Cartesian plane
- Pinpoint the x-axis and y-axis on a graph
- Recognize an intercept